Black Holes and the Golden Ratio

The golden ratio shows up in the physics of black holes!

Or does it?

Most things get hotter when you put more energy into them. But systems held together by gravity often work the other way. For example, when a red giant star runs out of fuel and collapses, its energy goes down but its temperature goes up! We say these systems have a negative specific heat.

The prime example of a system held together by gravity is a black hole. Hawking showed—using calculations, not experiments—that a black hole should not be perfectly black. It should emit ‘Hawking radiation’. So it should have a very slight glow, as if it had a temperature above zero. For a black hole the mass of the Sun this temperature would be just 6 × 10^-8 kelvin.

This is absurdly chilly, much colder than the microwave background radiation left over from the Big Bang. So in practice, such a black hole will absorb stuff—stars, nearby gas and dust, starlight, microwave background radiation, and so on—and grow bigger. But if we could protect it from all this stuff, and put it in a very cold box, it would slowly shrink by emitting radiation and losing energy, and thus mass. As it lost energy, its temperature would go up. The less energy it has, the hotter it gets: a negative specific heat! Eventually, as it shrinks to nothing, it should explode in a very hot blast.

But for a spinning black hole, things are more complicated. If it spins fast enough, its specific heat will be positive, like a more ordinary object.

And according to a 1989 paper by Paul Davies, the transition to positive specific heat happens at a point governed by the golden ratio! He claimed that in units where the speed of light and gravitational constant are 1, it happens when

$\displaystyle{ \frac{J^2}{M^4} = \frac{\sqrt{5} - 1}{2} }$

Here $J$ is the black hole’s angular momentum, $M$ is its mass, and

$\displaystyle{ \frac{\sqrt{5} - 1}{2} = 0.6180339\dots }$

is a version of the golden ratio! This is for black holes with no electric charge.

Unfortunately, this claim is false. Cesar Uliana, who just did a master’s thesis on black hole thermodynamics, pointed this out in the comments below after I posted this article.

And curiously, twelve years before writing this paper with the mistake in it, Davies wrote a paper that got the right answer to the same problem! It’s even mentioned in the abstract.

The correct constant is not the golden ratio! The correct constant is smaller:

$\displaystyle{ 2 \sqrt{3} - 3 = 0.46410161513\dots }$

However, Greg Egan figured out the nature of Davies’ slip, and thus discovered how the golden ratio really does show up in black hole physics… though in a more quirky and seemingly less significant way.

As usually defined, the specific heat of a rotating black hole measures the change in internal energy per change in temperature while angular momentum is held constant. But Davies looked at the change in internal energy per change in temperature while the ratio of angular momentum to mass is held constant. It’s this modified quantity that switches from positive to negative when

$\displaystyle{ \frac{J^2}{M^4} = \frac{\sqrt{5} - 1}{2} }$

In other words:

Suppose we gradually add mass and angular momentum to a black hole while not changing the ratio of angular momentum, $J,$ to mass, $M.$ Then $J^2/M^4$ gradually drops. As this happens, the black hole’s temperature increases until

$\displaystyle{ \frac{J^2}{M^4} = \frac{\sqrt{5} - 1}{2} }$

in units where the speed of light and gravitational constant are 1. And then it starts dropping!

What does this mean? It’s hard to tell. It doesn’t seem very important, because it seems there’s no good physical reason for the ratio of $J$ to $M$ to stay constant. In particular, as a black hole shrinks by emitting Hawking radiation, this ratio goes to zero. In other words, the black hole spins down faster than it loses mass.

Popularizations

Discussions of black holes and the golden ratio can be found in a variety of places. Mario Livio is the author of The Golden Ratio, and also an astrophysicist, so it makes sense that he would be interested in this connection. He wrote about it here:

• Mario Livio, The golden ratio and astronomy, Huffington Post, 22 August 2012.

Marcus Chown, the main writer on cosmology for New Scientist, talked to Livio and wrote about it here:

• Marcus Chown, The golden rule, The Guardian, 15 January 2003.

Chown writes:

Perhaps the most surprising place the golden ratio crops up is in the physics of black holes, a discovery made by Paul Davies of the University of Adelaide in 1989. Black holes and other self-gravitating bodies such as the sun have a “negative specific heat”. This means they get hotter as they lose heat. Basically, loss of heat robs the gas of a body such as the sun of internal pressure, enabling gravity to squeeze it into a smaller volume. The gas then heats up, for the same reason that the air in a bicycle pump gets hot when it is squeezed.

Things are not so simple, however, for a spinning black hole, since there is an outward “centrifugal force” acting to prevent any shrinkage of the hole. The force depends on how fast the hole is spinning. It turns out that at a critical value of the spin, a black hole flips from negative to positive specific heat—that is, from growing hotter as it loses heat to growing colder. What determines the critical value? The mass of the black hole and the golden ratio!

Why is the golden ratio associated with black holes? “It’s a complete enigma,” Livio confesses.

Extremal black holes

As we’ve seen, a rotating uncharged black hole has negative specific heat whenever the angular momentum is below a certain critical value:

$\displaystyle{ J < k M^2 }$

where

$\displaystyle{ k = \sqrt{2 \sqrt{3} - 3} = 0.68125003863\dots }$

As $J$ goes up to this critical value, the specific heat actually approaches $-\infty$ ! On the other hand, a rotating uncharged black hole has positive specific heat when

$\displaystyle{ J > kM^2}$

and as $J$ goes down to this critical value, the specific heat approaches $-\infty.$ So, there’s some sort of ‘phase transition’ at

$\displaystyle{ J = k M^2 }$

But as we make the black hole spin even faster, something very strange happens when

$\displaystyle{ J > M^2 }$

Then the black hole gets a naked singularity!

In other words, its singularity is no longer hidden behind an event horizon. An event horizon is an imaginary surface such that if you cross it, you’re doomed to never come back out. As far as we know, all black holes in nature have their singularities hidden behind an event horizon. But if the angular momentum were too big, this would not be true!

A black hole posed right at the brink:

$\displaystyle{ J = M^2 }$

is called an ‘extremal’ black hole.

Black holes in nature

Most physicists believe it’s impossible for black holes to go beyond extremality. There are lots of reasons for this. But do any black holes seen in nature get close to extremality? For example, do any spin so fast that they have positive specific heat? It seems the answer is yes!

Over on Google+, Robert Penna writes:

Nature seems to have no trouble making black holes on both sides of the phase transition. The spins of about a dozen solar mass black holes have reliable measurements. GRS1915+105 is close to $J=M^2.$ The spin of A0620-00 is close to $J=0.$ GRO J1655-40 has a spin sitting right at the phase transition.

The spins of astrophysical black holes are set by a competition between accretion (which tends to spin things up to $J=M^2$ ) and jet formation (which tends to drain angular momentum). I don’t know of any astrophysical process that is sensitive to the black hole phase transition.

That’s really cool, but the last part is a bit sad! The problem, I suspect, is that Hawking radiation is so pathetically weak.

But by the way, you may have heard of this recent paper—about a supermassive black hole that’s spinning super-fast:

• G. Risaliti, F. A. Harrison, K. K. Madsen, D. J. Walton, S. E. Boggs, F. E. Christensen, W. W. Craig, B. W. Grefenstette, C. J. Hailey, E. Nardini, Daniel Stern and W. W. Zhang, A rapidly spinning supermassive black hole at the centre of NGC 1365, Nature (2013), 449–451.

They estimate that this black hole has a mass about 2 million times that of our sun, and that

$\displaystyle{ J \ge 0.84 \, M^2 }$

with 90% confidence. If so, this is above the phase transition where it gets positive specific heat.

The nitty-gritty details

Here is where Paul Davies claimed the golden ratio shows up in black hole physics:

• Paul C. W. Davies, Thermodynamic phase transitions of Kerr-Newman black holes in de Sitter space, Classical and Quantum Gravity 6 (1989), 1909–1914.

He works out when the specific heat vanishes for rotating and/or charged black holes in a universe with a positive cosmological constant: so-called de Sitter space. The formula is pretty complicated. Then he set the cosmological constant $\Lambda$ to zero. In this case de Sitter space flattens out to Minkowski space, and his black holes reduce to Kerr–Newman black holes: that is, rotating and/or charged black holes in an asymptotically Minkowskian spacetime. He writes:

In the limit $\alpha \to 0$ (that is, $\Lambda \to 0$ ), the cosmological horizon no longer exists: the solution corresponds to the case of a black hole in asymptotically flat spacetime. In this case $r$ may be explicitly eliminated to give

$(\beta + \gamma)^3 + \beta^2 -\beta - \frac{3}{4} \gamma^2 = 0. \qquad (2.17)$

Here

$\beta = a^2 / M^2$

$\gamma = Q^2 / M^2$

and he says $M$ is the black hole’s mass, $Q$ is its charge and $a$ is its angular momentum. He continues:

For $\beta = 0$ (i.e. $a = 0$ ) equation (2.17) has the solution $\gamma = 3/4$ , or

$\displaystyle{ Q^2 = \frac{3}{4} M^2 } \qquad (2.18)$

For $\gamma = 0$ (i.e. $Q = 0$ ), equation (2.17) may be solved to give $\beta = (\sqrt{5} - 1)/2$ or

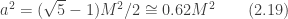
$\displaystyle{ a^2 = (\sqrt{5} - 1)M^2/2 \cong 0.62 M^2 } \qquad (2.19)$

These were the results first reported for the black-hole case in Davies (1979).

In fact $a$ can’t be the angular momentum, since the right condition for a phase transition should say the black hole’s angular momentum is some constant times its mass squared. I think Davies really meant to define

$a = J/M$

This is important beyond the level of a mere typo, because we get different concepts of specific heat depending on whether we hold $J$ or $a$ constant while taking certain derivatives!

In the usual definition of specific heat for rotating black holes, we hold $J$ constant and see how the black hole’s heat energy changes with temperature. If we call this specific heat $C_J,$ we have

$\displaystyle{ C_J = T \left.\frac{\partial S}{\partial T}\right|_J }$

where $S$ is the black hole’s entropy. This specific heat $C_J$ becomes infinite when

$\displaystyle{ \frac{J^2}{M^4} = 2 \sqrt{3} - 3 }$

But if instead we hold $a = J/M$ constant, we get something else—and this what Davies did! If we call this modified concept of specific heat $C_a$ , we have

$\displaystyle{ C_a = T \left.\frac{\partial S}{\partial T}\right|_a }$

This modified ‘specific heat’ $C_a$ becomes infinite when

$\displaystyle{ \frac{J^2}{M^4} = \frac{\sqrt{5}-1}{2} }$

After proving these facts in the comments below, Greg Egan drew some nice graphs to explain what’s going on. Here are the curves of constant temperature as a function of the black hole’s mass $M$ and angular momentum $J:$

The dashed parabola passing through the peaks of the curves of constant temperature is where $C_J$ becomes infinite. This is where energy can be added without changing the temperature, so long as it’s added in a manner that leaves $J$ constant.

And here are the same curves of constant temperature, along with the parabola where $C_a$ becomes infinite:

This new dashed parabola intersects each curve of constant temperature at the point where the tangent to this curve passes through the origin: that is, where the tangent is a line of constant $a=J/M.$ This is where energy and angular momentum can be added to the hole in a manner that leaves $a$ constant without changing the temperature.

As mentioned, Davies correctly said when the ordinary specific heat $C_J$ becomes infinite in another paper, eleven years earlier:

• Paul C. W. Davies, Thermodynamics of black holes, Rep. Prog. Phys. 41 (1978), 1313–1355.

You can see his answer on page 1336.

This 1978 paper, in turn, is a summary of previous work including an article from a year earlier:

• Paul C. W. Davies, The thermodynamic theory of black holes, Proc. Roy. Soc. Lond. A 353 (1977), 499–521.

And in the abstract of this earlier article, Davies wrote:

The thermodynamic theory underlying black-hole processes is developed in detail and applied to model systems. It is found that Kerr-Newman black holes undergo a phase transition at an angular-momentum mass ratio of 0.68M or an electric charge (Q) of 0.86M, where the heat capacity has an infinite discontinuity. Above the transition values the specific heat is positive, permitting isothermal equilibrium with a surrounding heat bath.

Here the number 0.68 is showing up because

$\displaystyle{ \sqrt{ 2 \sqrt{3} - 3 } = 0.68125003863\dots }$

The number 0.86 is showing up because

$\displaystyle{ \sqrt{ \frac{3}{4} } = 0.86602540378\dots }$

By the way, just in case you want to do some computations using experimental data, let me put the speed of light $c$ and gravitational constant $G$ back in the formulas. A rotating (uncharged) black hole is extremal when

$\displaystyle{ c J = G M^2 }$

This entry was posted on Thursday, February 28th, 2013 at 10:52 pm and is filed under astronomy, physics. You can follow any responses to this entry through the RSS 2.0 feed. You can leave a response, or trackback from your own site.

58 Responses to Black Holes and the Golden Ratio

Julien Haller says:

1 March, 2013 at 12:59 am

Fascinating! I’ve always been interested in the golden ratio’s relationship to aesthetics.

Sincerely,
Julien Haller

Reply
Simon Willerton says:

1 March, 2013 at 8:14 am

With a title like “Black Holes and The Golden Ratio” I suspected you were going to discuss an article from Chaos, Solitons & Fractals.

Reply
- John Baez says:
  
  1 March, 2013 at 9:30 pm
  
  If the editor of that esteemed journal were still in business, we could expect some papers on this subject in a couple of weeks. I really got the feeling he was publishing some papers based on combinations of ideas in This Week’s Finds.
  
  Alas, we’ll have to content ourselves with this:
  
  • Lan Xu and Ting Zhong, Golden ratio in quantum mechanics, Nonlinear Science Letters B: Chaos, Fractal and Synchronization (2011), 24.
  
  Abstract. The experimental discovery of the golden mean in quantum mechanics by the Helmholtz group (R. Coldea, et al., Golden ratio discovered in a quantum world, Science, 8 January, 2010) is discussed. The direct and important relation of this discovery with El Naschie’s E-infinity theory is outlined.
  
  Keywords: Golden mean, experiment at Helmholtz Centre, E-infinity theory, quantum mechanics.
  
  The experimental discovery of the golden ratio in quantum magnetism [1] is an extremely important milestone in the quest for the understanding of quantum mechanics and E-infinity theory. We full heartedly agree with the explanation and discussion given by Prof. Affleck [2] is concerned. For this reason we would like to draw attention to a general theory dealing with the noncommutativity and the fine structure of spacetime which comes to similar conclusions and sweeping generalizations about the important role which the golden mean must play in quantum and high energy physics. Maybe the most elementary way to explain this point of view is the following: Magnetism is just one aspect of the five fundamental forces of nature. In a unified picture where all the five forces melt into one it is reasonable to suspect that the golden ratio will play a fundamental role. This fact immediately follows from the work of the French mathematician Alain Connes and the Egyptian engineering scientist and theoretical physicist M.S. El Naschie. In Connes’ noncommutative geometry his dimensional function is explicitly dependant on the golden mean. Similarly the bijection formula in the work of El Naschie is identical with this dimensional function and implies the existence of random Cantor sets with golden mean Hausdorff dimension as the building blocks of a spacetime which is a Cantor set-like fractal in infinite dimensional but hierarchal space. Invoking Albert Einstein’s ideas connecting spacetime to geometry with energy and matter, it is clear that these golden mean ratios must appear again in the mass spectrum of elementary particles and other constants of nature. There are several places where this work can be found [3,4,5].
  
  That’s not just the abstract. That’s the whole paper!
  
  Reply
  - Bradley Robinson says:
    
    2 March, 2013 at 2:00 am
    
    Lots I barely understand here and apologies if my questions are uninformed or irrelevant but what is the fifth force of nature… Ok..I googled it… I am disappointed that the comments indicate conflicting results but the general thread resonates with what I would like to keep in mind. My intuition begs me to ask if the reciprocal of gravity could be described as compression?
    
    Reply
Arrow says:

1 March, 2013 at 8:49 am

“Most physicists believe it’s impossible for black holes to go beyond extremality.”

But are there any arguments for that beyond the fact that naked singularity makes people uneasy? (Of course I also find the possibility of a physically real, naked, or otherwise, singularity implausible but I see it as a limitation of the theory, not a law black holes have to obide.)

Reply
- Cesar Uliana says:
  
  1 March, 2013 at 10:42 pm
  
  Actually, a lot is known on this subject. Israel proved in the 80s that if your black hole is sub-extremal it cannot get extremal in a finite time (in a proper sense of finite time). So if you want to make a naked singularity you woul have to go from sub- to super-extremal without passing trought extremality.
  
  That quite recently became a hot subject, and in fact there are suggestions that one could use quantum mechanics to throw a particle in a near extremal black hole and produce a, probably, naked singularity. But I think that without violating energy conditions (which are obeyed in classical mechanics) there is abundant numerical evidence that you always gets less angular momentum in than mass, so you are always stuck with the black hole
  
  Reply
Cesar Uliana says:

1 March, 2013 at 12:41 pm

Very interesting and somewhat wrong it appears. The value you quote disagrees with the 1979 paper. In fact by looking at the original paper,

• P. C. W. Davies, The thermodynamic theory of black holes, Proc. R. Soc. Lond. A 353 (1977), 499-521.

one sees that the value for the Reissner-Nordstrom agrees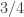 $3/4$ , but the one for the Kerr is actually 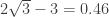$2\sqrt{3}-3=0.46$ . If you start with the Kerr metric is easy to evalute the specific heat and show that the original value is the correct one, and the golden ratio is a mistake in the subsequent paper. Not sure where the error came from though, specially considering that RN came out right in both papers. I’m thinking something went awry when he took the limit 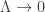$\Lambda\rightarrow 0$ , but not sure. I’ll try to take a better look later and see what I get.

Reply
- John Baez says:
  
  1 March, 2013 at 9:38 pm
  
  That sounds pretty weird, given that Davies wrote both papers. Why would he get the right answer in the first paper and a false but very exciting answer in the second one? Given how attention-grabbing the golden ratio is, it’s hard to imagine him overlooking the fact that he got a different answer the first time!
  
  Thanks for pointing this out. I hope you can help me get to the bottom of this. I’m not working on quantum gravity anymore, so I have no excuse for spending much time on this puzzle, but it’s intriguing. I’ll start by taking a peek at the 1977 paper, and the 1979 paper. (I assume that’s a different paper?)
  
  Reply
- Cesar Uliana says:
  
  1 March, 2013 at 10:30 pm
  
  I’ve just finished my masters thesis on black hole thermodynamics, and having written the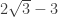 $2\sqrt{3}-3$ result it came to me as a shock when you mentioned the golden ratio. At first I hoped very strongly that I made a mistake, because it would be a lot more nice to have a golden ratio, but could not find any in my notes.
  
  The 1977 and 1979 papers are different, the 1977 is all about equation of state for Kerr-Newman and it is more explicit on the phase transition. The 1979 is more like a review on BH thermodynamics, with the semiclassical effects and everything, and just quotes the previous paper on the critical values.
  
  It is very bizarre indeed that Davies could overlook such thing, I’ll try to rework $C_J$ both starting from Kerr and Kerr-deSitter this weekend to see if I can see what is going on. As soon as possible I’ll come back here with whatever I find. Thanks for the attention.
  
  Reply
  - John Baez says:
    
    1 March, 2013 at 11:09 pm
    
    Thanks for your attention! It’s a case where getting the right reader—the one who actually knows what’s going on—makes a huge difference! Let me know how it goes. I’ll look at those papers myself.
    
    Reply
Gabe says:

1 March, 2013 at 1:35 pm

Could the phase transition point be related to the distribution of galaxies?

Accretions below the phase point end up not becoming supermassive, dissipate. Above, become attractors and then galactic centres.

Measure the distribution of stars that can become black holes vs other less dense remnants. Any golden ratios in there?

Reply
imbuteria says:

1 March, 2013 at 4:10 pm

Reblogged this on Imbuteria's Blog.

Reply
amarashiki says:

1 March, 2013 at 4:33 pm

Amazing. Personally, I think it means that, maybe, there is a maximum angular momentum to avoid “naked singularities”. Otherwise, we should understand what IS the meaning and proper way to “live with” naked singularities. Black holes are yet a “black box” to be decyphered…

Reply
Uwe Stroinski says:

1 March, 2013 at 4:42 pm

Maybe this is pedantic, but the number you use in the text is not the golden ratio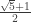 $\frac{\sqrt{5}+1}{2}$ .

Reply
- Todd Trimble says:
  
  1 March, 2013 at 8:22 pm
  
  It could of course be considered a matter of taste: is it 1.618… or its reciprocal .618… that is the golden ratio? But you’re probably right, that for most people it would be $\overset{\lim}{n \to \infty} \frac{F_{n+1}}{F_n}$ . Wikipedia agrees with you.
  
  Reply
- John Baez says:
  
  1 March, 2013 at 9:17 pm
  
  I usually use the phrase ‘golden ratio’ to mean
  
  $\displaystyle{ \frac{\sqrt{5} + 1}{2} = 1.6180339.... }$
  
  but I’ve seen that term used almost equally often to refer to its reciprocal,
  
  $\displaystyle{ \frac{\sqrt{5}-1}{2} = 0.6180339... }$
  
  So, I thought for the purposes of this article I’d go along with that other convention.
  
  I don’t trust the Wikipedia article to have its finger on the pulse of this situation, because it claims that the bigger of these numbers is called $\phi$ and the smaller one is called $\Phi.$ Besides the illogicality of using the bigger letter to stand for the smaller number, I’ve mainly seen them used the opposite way!
  
  They also say the smaller one is called ‘the golden number conjugate’… and while I understand the logic of this, coming from the general theory of algebraic number fields, I’ve never seen that phrase in use.
  
  Reply
  - zawy (@zawy3) says:
    
    25 September, 2017 at 11:22 am
    
    Wiki defines the golden ratio as a/b that solves the equation a/b = (a+b)/a which gives 1.618 and -0.618. It comes from a simple geometrical expression so I’d say this is the best definition. Don’t forget meters = i * c * seconds (“Relativity” app 2 which allows Occam-required reduction from Minkowski space to Euclidean space) so don’t throw away the negative in these considerations.
    
    Reply
John Baez says:

2 March, 2013 at 12:21 am

In case anyone is interested, I’ve found a freely available version of the paper where Davies claims the golden ratio shows up. I’ve added this discussion of it to my blog entry:

• Paul Davies, Thermodynamic phase transitions of Kerr-Newman black holes in de Sitter space, Classical and Quantum Gravity 6 (1989), 1909.

He works out when the specific heat vanishes for rotating and/or charged black holes in a universe with a positive cosmological constant: so-called de Sitter space. The formula is pretty complicated. Then he takes the limit where the cosmological constant goes to zero, de Sitter space flattens out to Minkowski space, and his black holes reduce to Kerr–Newman black holes: that is, rotating and/or charged black holes in an asymptotically Minkowskian spacetime. He writes:

In the limit $\alpha \to 0$ (that is, $\Lambda \to 0$ ), the cosmological horizon no longer exists: the solution corresponds to the case of a black hole in asymptotically flat spacetime. In this case $r$ may be explicitly eliminated to give

$(\beta + \gamma)^3 + \beta^2 -\beta - \frac{3}{4} \gamma^2 = 0. \qquad (2.17)$

Here

$\beta = a^2 / M^2$

$\gamma = Q^2 / M^2$

and he says $M$ is the black hole’s mass, $Q$ is its charge and $a$ is its angular momentum. He continues:

For $\beta = 0$ (i.e. $a = 0$ ) equation (2.17) has the solution $\gamma = 3/4$ , or

$\displaystyle{ Q^2 = \frac{3}{4} M^2 } \qquad (2.18)$

For $\gamma = 0$ (i.e. $Q = 0$ ), equation (2.17) may be solved to give $\beta = (\sqrt{5} - 1)/2$ or

$\displaystyle{ a^2 = (\sqrt{5} - 1)M^2/2 \cong 0.62 M^2 } \qquad (2.19)$

These were the results first reported for the black-hole case in Davies (1979).

In fact it seems $a$ can’t be the angular momentum, since the right condition for a phase transition should say the black hole’s angular momentum is some constant times its mass squared. I think he really meant to define $a = J/M.$

Reply
Boris Borcic says:

2 March, 2013 at 1:41 am

Fun post! Doesn’t the “phase transition” mean there is a 1D line or curve in Kerr-Newman parameter space relating all BH at the transition, and such that, concievably, lower mass BH on the line could be fed to larger mass while keeping them on the critical line… isothermically??

What’s the common phase transition temperature, in that case?

Reply
- John Baez says:
  
  2 March, 2013 at 1:55 am
  
  Hmm, the space of Kerr–Newman solutions has points given by triples $(M,J,Q)$ : mass, angular momentum and charge. The so-called ‘phase transition’ we’re talking about is the surface where the specific heat is infinite. This means that if $E$ is energy and $T$ is temperature,
  
  $\displaystyle{ \frac{\partial T}{\partial E} = 0 }$
  
  or if you relate the black hole’s energy to its mass,
  
  $\displaystyle{ \frac{\partial T}{\partial M} = 0 }$
  
  Is this what you meant by ‘isothermic’?
  
  Reply
  - John Baez says:
    
    2 March, 2013 at 4:05 am
    
    Hmm, a paper I’m reading seems to say the right quantity to think about when defining specific heat (or ‘heat capacity’) is not $E$ but the ‘internal energy’ $U,$ given by
    
    $U = E - \Omega_H J$
    
    where $\Omega_H$ is the angular velocity of the horizon. This is a way of subtracting off the rotational energy of the black hole.
    
    Reply
  - Boris Borcic says:
    
    2 March, 2013 at 10:04 am
    
    By “isothermic” I meant “while keeping temperature constant”, but the reasoning was certainly more intuitive with a zero rather than infinite specific heat at the transition (you hadn’t been very specific we were in the latter case).
    
    Reply
    - John Baez says:
      
      2 March, 2013 at 4:29 pm
      
      Boris wrote:
      
      (you hadn’t been very specific we were in the latter case).
      
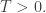      Yes, I wasn’t very clear about that, and I’ll fix my blog article to make it more clear. This makes the term ‘phase transition’ more justified. But ‘phase transition’ may remind you of melting ice, where it takes a positive amount of energy to increase the temperature from any $T < 0$ to any $T > 0.$ This is quite different, because there’s not a ‘jump discontinuity’ in the energy, as with ice… just a surface in the phase space of a Kerr black hole such that the specific heat becomes infinitely big and positive as we approach this surface from one side, and infinitely big and negative as we approach it from the other.
      
      This can be seen from the formula for the specific heat:
      
      $C = \displaystyle{ \frac{2 \pi \left(r_--r_+\right) r_+ \left(r_-+r_+\right){}^2}{-3 r_-^2-6 r_+ r_-+r_+^2} }$
      
      where
      
      $\displaystyle{ M = \frac{r_+ + r_-}{2} }$
      
      and
      
      $\displaystyle{ \frac{J}{M} = \sqrt{r_+ r_-} }$
John Baez says:

2 March, 2013 at 3:59 am

I’m trying to recheck the calculation, starting not from first principles but from this:

• Jianyong Shen, Rong-Gen Cai, Bin Wang and Ru-Keng Su, Thermodynamic geometry and critical behavior of black holes.

Here’s what they say. In Planck units, the specific heat for a Kerr black hole blows up when

$r_+^2 - 6 r_+ r_- - r_-^2 = 0$

where $r_+, r_-$ are the radii of the outer and inner horizons. These are related to the mass $M$ and angular momentum $J$ by

$\displaystyle{ M = \frac{r_+ + r_-}{2} }$

and

$\displaystyle{ \frac{J}{M} = \sqrt{r_+ r_-} }$

These equations are quite pretty! And they should let us determine, for a given $M,$ which $J$ gives infinite specific heat. But annoyingly, I’m not getting either

$J^2 = \displaystyle{ \frac{\sqrt{5} -1}{2} } M^4$

or

$J^2 = (2 \sqrt{3} - 3) M^4$

I could be making a dumb algebra mistake, or reading their paper wrong. Can someone check?

They call this “the phase transition point of a Kerr black hole given by Davies.” They cite all three of his papers, without any comment on the fact that they give different answers to the puzzle here.

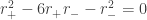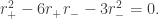[Moderator’s note: the formula $r_+^2 - 6 r_+ r_- - r_-^2 = 0$ turns out to be wrong; see below. It should be $r_+^2 - 6 r_+ r_- - 3r_-^2 = 0.$ ]

Reply
Greg Egan says:

2 March, 2013 at 7:39 am

There’s an error in equation (21) of Jianyong Shen, Rong-Gen Cai, Bin Wang and Ru-Keng Su, Thermodynamic geometry and critical behavior of black holes: the factor of 2 in the denominator shouldn’t be there!

I haven’t followed the consequences in their subsequent calculations of the curvature of the thermodynamic manifold, so I’m not sure if that error alone is the cause of the discrepancy, but from a much simpler calculation I get:

$\displaystyle{ \left(\frac{\partial S}{\partial T}\right)_J = -\frac{16 \pi ^2 M^3 \left(J^4+3 J^2 M^4-6 M^6 \left(\sqrt{M^4-J^2}+M^2\right)\right)}{J^4+6 J^2 M^4-3 M^8} }$

which should be proportional to the specific heat at constant J, and the denominator has a zero when:

$J^2 = (2\sqrt{3} - 3) M^4$

Reply
Greg Egan says:

2 March, 2013 at 7:53 am

For the actual heat capacity at constant J, the result I get is almost the same as their equation (27), but there’s a coefficient of -3 rather than -1 for the polynomial in the denominator, giving:

$\displaystyle{ C_J = \frac{2 \pi \left(r_--r_+\right) r_+ \left(r_-+r_+\right){}^2}{-3 r_-^2-6 r_+ r_-+r_+^2} }$

Reply
- John Baez says:
  
  2 March, 2013 at 4:11 pm
  
  Great! I figured it must be a mistake of theirs, since I rechecked my own calculation a depressingly large number of times… but it was after a day of grading 60 homework sets of 12 problems each, so I thought my brain might have malfunctioned. That 3 should save the day.
  
  Reply
Greg Egan says:

2 March, 2013 at 12:30 pm

I think it’s possible to give an almost self-contained computation of the heat capacity in just a few lines, if we take for granted the area of a Kerr black hole in terms of its mass and angular momentum:

$\displaystyle{ A = 8\pi (M^2 + \sqrt{M^4-J^2}) }$

That’s from equation (278) in Chandrasekhar’s The Mathematical Theory of Black Holes. We then have the entropy equal to a quarter of this, in suitable units:

$\displaystyle{ S = \frac{A}{4} = 2\pi (M^2 + \sqrt{M^4-J^2}) }$

If we follow Jianyong Shen et al. and write conservation of energy as:

$dM = T dS + \Omega_H dJ$

it follows that:

$\displaystyle{ T = \left(\frac{\partial M}{\partial S}\right)_J = 1 / \left(\frac{\partial S}{\partial M}\right)_J }$

We’ll shortly need a result we can derive from this easily:

$\displaystyle{ \left(\frac{\partial T}{\partial M}\right)_J = -\left(\frac{\partial^2 S}{\partial M^2}\right)_J / \left(\frac{\partial S}{\partial M}\right)_J^2 }$

Now, heat capacity with any set of variables held constant can be found by taking the derivative of the entropy with respect to T, holding those same variables constant, and then multiplying by T (Reif’s Fundamentals of Statistical and Thermal Physics, equation (4.4.8)). So we have:

$\displaystyle{ C_J = T \left(\frac{\partial S}{\partial T}\right)_J }$

where

$\displaystyle{ \left(\frac{\partial S}{\partial T}\right)_J = \left(\frac{\partial S}{\partial M}\right)_J / \left(\frac{\partial T}{\partial M}\right)_J = -\left(\frac{\partial S}{\partial M}\right)_J^3 / \left(\frac{\partial^2 S}{\partial M^2}\right)_J }$

So we have simply:

$\displaystyle{ C_J = -\left(\frac{\partial S}{\partial M}\right)_J^2 / \left(\frac{\partial^2 S}{\partial M^2}\right)_J = \frac{4 \pi M^2 \left(M^2 \sqrt{M^4-J^2}-J^2+M^4\right)}{-2 M^2 \sqrt{M^4-J^2}+J^2+M^4} }$

or if we define $j=J^2/M^4$ , we have:

$\displaystyle{ C_J = \frac{4 \pi \left(-j+\sqrt{1-j}+1\right) M^2}{j-2 \sqrt{1-j}+1} }$

The denominator is zero at:

$j = J^2/M^4 = 2\sqrt{3}-3$

Reply
- John Baez says:
  
  2 March, 2013 at 4:31 pm
  
  Bravo!
  
  Reply
Cesar Uliana says:

2 March, 2013 at 5:20 pm

Ok, so thanks to Greg Egan who patiently did the whole Kerr case. I’ve double checked this today with my previous notes and it all agrees, which is great. So it remains to discover how come Davies got it wrong in 1989.

Reworking this paper everything seems ok until equations (2.11) and (2.12) which are

$3\alpha r^6+(1+8\alpha\beta)r^4+(\alpha\beta^2-4\beta-3\gamma)r^2-\beta(\beta+\gamma)=0$

and

$\beta=(2r-\gamma)/(1-\alpha r^2) -r^2$

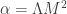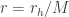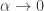where $\alpha=\Lambda M^2$ and $r=r_h/M$ . The first equation is $C_J=0$ and the second one is the constraint $\Delta=0$ , which in this case imposes that the radius is the one for the event horizon. This equations are correct as far as I have not made any mistake. Then doing some algebra and taking $\alpha\rightarrow 0$ one gets (2.17), the cubic equation on the post. This one results both in the correct answer for the Reissner-Nordstrom case and the wrong one for the Kerr as Greg Egan kindly pointed out above.

Fine, so what now? If one is brave enough, one should solve the second equation, which is quartic, for $r$ and find a rational function $r(\beta)$ . Then one puts the answer (assuming one can see which is the second largest root as Davies points out) in the first equation, then solves for $\beta$ to find the parameters where $C_J$ diverges. That is not what Davies does. He puts $\alpha=0$ and then proceeds to simplify.

But that is really a problem, becaue $\alpha$ is the parameter that controls the largest exponent of $\beta^n$ in the first equation. So is kind of dangerous to put it to zero as one can see in a simple example

$ax^2+bx+c$

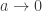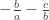with roots $-\frac{b}{2a}\pm\frac{b}{2a}\sqrt{1-\frac{4ac}{b^2}}$ . If one tries to take $a\rightarrow 0$ then one could taylor expand the square root obtaining $-\frac{c}{b}$ (the correct answer) and $-\frac{b}{a}-\frac{c}{b}$ which diverges. That ilustrates the risks in taking the parameter in front of the largest exponent to zero, this limit is not well behave at all. In the black hole case is hard to tell (at least for me) if that is the case, because since the equation is of order 6 I can’t probe what is going on. But I’m positive by this discussion that one should not trust this limit. Of course it will spit out the correct answer eventually (as it does for RN) but is hard to see where the correct answer went. I suppose the value 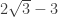$2\sqrt{3}-3$ was “trapped” in a side that diverged and did not appear in the simplified equation with $\alpha=0$ , though I’m not sure if that could be the case. I tried to look at cubic equations for this behavior but it gets very messy quickly.

So definitely not answering what is wrong, but maybe a hint. I’ll be glad if anyone could point any errors, or further suggestions for a solution with feasible algebra.

Reply
Greg Egan says:

2 March, 2013 at 11:58 pm

John mentioned already that what Davies calls the angular momentum, a, is really an angular momentum parameter, J/M. In Davies’ 1989 paper he talks about the specific heat at constant values of the cosmological constant, charge, and a. But $C_a \neq C_J$ ; the two involve holding different things constant!

That still doesn’t bring in the golden ratio, though; I get:

$\begin{array}{ccl} C_a &=& \displaystyle{ -\frac{2 \pi \sqrt{M^2-a^2} \left(2 M \left(\sqrt{M^2-a^2}+M\right)-a^2\right)}{2 \sqrt{M^2-a^2}-M} } \\ \\ &=& \displaystyle{ \frac{2 \pi \sqrt{M^4-J^2} \left(2 M^2 \left(\sqrt{M^4-J^2}+M^2\right)-J^2\right)}{M^4-2 M^2 \sqrt{M^4-J^2}} } \end{array}$

which is infinite when:

$J^2/M^4 = 3/4$

But it’s worth keeping in mind that even if we figure out how to take the limit correctly for the charge and cosmological constant going to zero, if we do that with an equation of Davies that was derived by holding a rather than J constant, we won’t get $C_J$ !

[Moderator’s note: there’s a mistake here, which Egan corrects below.]

Reply
- John Baez says:
  
  3 March, 2013 at 1:24 am
  
  Good point. I’ll try to improve some remarks in my blog entry. I’m taking the liberty of continually updating this entry so it provides the most correct story I can manage at a given time, without sounding any wiser than I actually am… since I figure that in future, most people won’t read all the comments, and the myth of ‘black holes and the golden ratio’ had already propagated a bit, before I got involved, so I want to squelch it as effectively as I can.
  
  (I’ve also added links to a couple of news articles from 2003 and 2012, which had spread this mistake.)
  
  Reply
- Greg Egan says:
  
  3 March, 2013 at 2:13 am
  
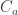  Oops, my $C_a$ above is wrong; I was assuming I could just re-express the entropy as a function of a and M rather than J and M and then use exactly the same final formula for the specific heat in terms of derivatives of the entropy, holding a constant rather than J. But that gives the wrong temperature in the intermediate steps. I’ll do this more carefully, but I thought I’d better get in quickly and retract the mistake!
  
  Reply
Greg Egan says:

3 March, 2013 at 4:03 am

Ah, mystery solved!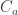 $C_a$ does go to infinity at the golden ratio, even though $C_J$ doesn’t!

I redid the calculation for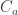 $C_a$ using the correct temperature (my apologies for the mistaken first attempt above).

The entropy can be expressed either as:

$\displaystyle{S(M,J) = 2 \pi \left(\sqrt{M^4-J^2}+M^2\right)}$

or as:

$\displaystyle{S(M,a) = 2 \pi M \left(\sqrt{M^2-a^2}+M\right)}$

where the angular momentum parameter $a$ is defined as:

$a = J / M$

The temperature is:

$\displaystyle{T = 1/\left(\frac{\partial S(M,J)}{\partial M}\right)_J = \frac{\sqrt{M^4-J^2}}{4 \pi M \left(\sqrt{M^4-J^2}+M^2\right)}}$

which we can reexpress in terms of $a$ as:

$\displaystyle{T = \frac{\sqrt{M^2-a^2}}{4 \pi M \left(\sqrt{M^2-a^2}+M\right)}}$

It follows that:

$\displaystyle{\sqrt{M^2-a^2} (1-4 \pi M T)-4 \pi M^2 T = 0}$

Differentiating this with respect to $T$ while holding $a$ constant lets us compute:

$\displaystyle{\left(\frac{\partial M}{\partial T}\right)_a = \frac{4 \pi M^2 \left(M^3 \left(\sqrt{M^2-a^2}+M\right)-a^4\right)}{a^4+a^2 M^2-M^4}}$

The specific heat we want this time is:

$\displaystyle{C_a = T \left(\frac{\partial S}{\partial T}\right)_a}$

Making use of our expression for $\left(\frac{\partial M}{\partial T}\right)_a$ this turns out to be:

$\displaystyle{C_a = -\frac{2 \pi M \left(a^2 M^3-2 M^4 \left(\sqrt{M^2-a^2}+M\right)+a^4 \left(\sqrt{M^2-a^2}+M\right)\right)}{a^4+a^2 M^2-M^4}}$

or in terms of $J$ rather than $a$ :

$\displaystyle{C_a = -\frac{2 \pi \left(J^2 M^6-2 M^8 \left(\sqrt{M^4-J^2}+M^2\right)+J^4 \left(\sqrt{M^4-J^2}+M^2\right)\right)}{J^4+J^2 M^4-M^8}}$

The denominator now has a zero when:

$J^2/M^4 = \frac{1}{2} \left(\sqrt{5}-1\right)$

Reply
Cesar Uliana says:

3 March, 2013 at 4:20 am

Fantastic, I’m thrilled that the golden ratio was indeed there. At first I would like to apologize for my sloppy mistake in confusing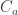 $C_a$ with $C_J$ when looking at the papers from Davies, and congrats to Greg Egan for realising that and for the explicit formulas which at last justify the title in the post.

Reply
Greg Egan says:

3 March, 2013 at 7:50 am

I guess the remaining question is whether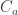 $C_a$ is in any sense as natural or physically relevant as $C_J$ . It seems more natural to me to ask what happens to the temperature of a spinning black hole as you drop matter into it that has zero angular momentum at infinity, than to drop matter in that has exactly the angular momentum needed to keep $a=J/M$ constant.

Reply
- Boris Borcic says:
  
  3 March, 2013 at 9:28 am
  
  This stands out as an enlightening summary for who’s divorced from equations. I am not clear at what exact mixture of science, fiction, and pedagogy the thought becomes legitimate, but what I get from the thread is that perhaps teachers need to declare black holes as a concept, “the wormhole into mathematical space”.
  
  What’s a contorted way to say I the post and thread leaves me yearning for a couple more graphics:)
  
  Reply
  - Boris Borcic says:
    
    3 March, 2013 at 10:40 am
    
    Oops, read the end “the post and thread leave us yearning for more plots”.
    
    Perhaps not just a couple plots, though – a graphic BH explorer not about simulating single BH encounters, but meant for creating coordinated plots across parameter (phase?) space. A learning environment that would teach BH through teaching how to define the plots. That would sure be cool.
    
    Reply
- John Baez says:
  
  3 March, 2013 at 4:53 pm
  
  Congratulations for getting further to the bottom of this! The usual specific heat $C_J$ seems more important to me than $C_a,$ and nothing suggests to me that the golden ratio is showing up here for a ‘profound’ reason—one that would shed light on something if we could dig even deeper. But at least you’ve clarified what’s going on. With luck, this story will sit here until the collapse of our current civilization, and anyone can read it and try to come up with a good idea. If I were trying to ‘rescue’ this appearance of the golden ratio, I’d start by pondering $a = J/M.$
  
  Reply
  - Boris Borcic says:
    
    3 March, 2013 at 6:56 pm
    
    How does BH evaporation drive J/M ?
    
    Reply
- John Baez says:
  
  3 March, 2013 at 9:04 pm
  
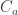  Over on Google+, Boris Borcic said it would be nice if Hawking radiation from a rotating uncharged black hole carried away energy and angular momentum at a rate that leaves $a = J/M$ constant. This could make the quantity $C_a$ physically interesting.
  
  Maybe Cesar knows the formulas for the rates at which Hawking radiation changes $M$ and $J$ for a rotating uncharged black hole.
  
  Reply
Greg Egan says:

3 March, 2013 at 11:31 pm

Regarding Boris Borcic’s comment on Google Plus wondering if maybe J/M is preserved by Hawking radiation, I think the answer is that angular momentum is radiated away faster than the mass.

I wish I could find a clear reference for this, but all the papers I’ve found on the subject on the arXiv are about higher-dimensional black holes created in particle accelerators! They all mention a “spin-down” phase followed by a “Schwarzschild” phase, i.e. the loss of most angular momentum well before the hole itself evaporates. And it sounds like this is not some special higher-dimensional phenomenon in contrast to the usual case.

I suspect the answer for ordinary (3+1)-dimensional black holes can be found in this really early paper on the subject: D. N. Page, “Particle Emission Rates From A Black Hole. 2. Massless Particles From A Rotating Hole”, Phys. Rev. D 14 (1976) 3260, but I don’t think it’s available online and I’m not in a position to be able to consult a library copy in the immediate future.

Reply
- John Baez says:
  
  4 March, 2013 at 3:07 am
  
  Yes, like you I was only easily to find stuff on higher-dimensional black holes in braneworld scenarios… clearly from the good old days when extreme optimists were hoping to see them created by the Large Hadron Collider, thus vindicating string theory.
  
  I’ll try to get ahold of the paper you mention, but right this moment my virtual private network to UC Riverside is broken for some reason.
  
  I also decided to cash in some chips and ask Ashtekar about this.
  
  Reply
- Blake Stacey says:
  
  4 March, 2013 at 2:50 pm
  
  Page’s 1976 paper is available online, but only if you have an APS subscription (grumble, grumble). The paper to which it is a sequel, available (for now) for free, discusses spin-down in the context of justifying why it’s reasonable to study the nonrotating case at length.
  
  Reply
Greg Egan says:

4 March, 2013 at 3:07 am

I found the abstract of Page’s 1976 paper online, and it confirms that rotating black holes spin down as they radiate, i.e. J goes to zero faster than M.

Particle emission rates from a black hole. II. Massless particles from a rotating hole

Don N. Page W. K. Kellogg Radiation Laboratory, California Institute of Technology, Pasadena, California 91125

The calculations of the first paper of this series (for nonrotating black holes) are extended to the emission rates of massless or nearly massless particles from a rotating hole and the consequent evolution of the hole. The power emitted increases as a function of the angular momentum of the hole, for a given mass, by factors of up to 13.35 for neutrinos, 107.5 for photons, and 26 380 for gravitons. Angular momentum is emitted several times faster than energy, so a rapidly rotating black hole spins down to a nearly nonrotating state before most of its mass has been given up. The third law of black-hole mechanics is proved for small perturbations of an uncharged hole, showing that it is impossible to spin up a hole to the extreme Kerr configuration. If a hole is rotating fast enough, its area and entropy initially increase with time (at an infinite rate for the extreme Kerr configuration) as heat flows into the hole from particle pairs created in the ergosphere. As the rotation decreases, the thermal emission becomes dominant, drawing heat out of the hole and decreasing its area. The lifetime of a black hole of a given mass varies with the initial rotation by a factor of only 2.0 to 2.7 (depending upon which particle species are emitted). If a nonrotating primordial black hole with initial mass 5 × 10^14 g would have just decayed away within the present age of the universe, a hole created maximally rotating would have just died if its initial mass were about 7 × 10^14 g. Primordial black holes created with larger masses would still exist today, but they would have a maximum rotation rate determined uniquely by the present mass. If they are small enough today to be emitting many hadrons, they are predicted to be very nearly nonrotating.

Reply
- Cesar Uliana says:
  
  4 March, 2013 at 2:55 pm
  
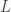  Hum, I’ve been looking at this paper, and the rate of change for $J$ is really gigantic, not sure if one can derive much information from it, though if you want I can reproduce it here. But one could get and idea by noting that any process in a black hole that changes the mass by $E$ and the angular momentum by $L$ must satisfy $E>\Omega L$ , where $\Omega=\frac{a}{r_+^2+a^2}$ is the black hole angular velocity and $r_+ = \sqrt{A/4\pi}$ is the event horizon radius. Since $\Omega$ is always defined as to be positive one can see that in general any process will change the angular momentum more than the mass, but surely one could come up with a process where $a$ is constant, it does not defy any law. If one looks at Hawking radiation for fields in nature as Page suggests is easy to see that for non-scalar fields you have radiation with arbitrarily small energy and fixed angular momentum that carries the spin out of the black hole faster than mass, thus leading to Schwarzschild asymptotically. I can’t find the right reference now, but I recall a seminar where the speaker told me about a result for scalar fields where the $l=0$ modes contribute, and thus for the Kerr you had the situation where you could extract mass without changing the angular momentum. But since the rates have different functional forms for different spin of the fields I would guess it is not possible to create a field configuration where the s-wave modes of the scalar field balance every other contribution so as to the black hole lose mass but at fixed $a$ .
  
  Even if one could come up with some way of Hawking radiating at fixed $a$ is would be hard to maintain because Hawking radiation must satisfy $\frac{m}{\omega}<\frac{1}{\Omega}$ , where $m$ is the azimuthal number and $\omega$ the frequency. If you want $a$ fixed then you the ratio should be constant. But as the black hole radiates the mass, and the area, decrease, which increases the angular velocity $\Omega$ and then it would reduce the number of configurations with $\frac{m}{\omega}<\frac{1}{\Omega}$ where the radiation occurs. Definitely not impossible, just hard I guess.
  
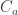  Regarding the difference between $C_J$ and $C_a$ , the question is what possible physical significance could 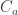$C_a$ have. At fixed angular momentum is the specific heat with no work done, so it satisfies Maxwell's relation for the second derivatives as well as all the usual stuff from thermodynamics such as that relation 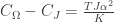$C_\Omega -C_J = \frac{TJ\alpha^2}{K}$ where $f$ is a function of the thermal expansion $\alpha$ and the compressibility $K$ . 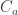I had never seen $C_a$ being discussed before, hence my surprise, and is hard to see what could it mean for physical process, but I'm glad I was entirely mistaken.
  
  Reply
  - davetweed says:
    
    4 March, 2013 at 8:52 pm
    
    Cesar wrote
    
    … Hawking radiation must satisfy $\frac{m}{\omega}<\frac{1}{\Omega}$ , where m is the azimuthal number…
    
    Is this a clue to the reasons for the name of the blog? That we’ll accumulate so much information that its embodiment will ultimately be so massive it becomes a black hole, accessible only by descrambling its Hawking radiation. (Only joking. I think.)
    
    Reply
  - John Baez says:
    
    4 March, 2013 at 9:08 pm
    
    I mainly chose the name Azimuth because it sounds cool and it doesn’t have strong associations: most people can’t remember what it means. But it comes from from Arabic as-sumut “the ways,” plural of as-samt “the way, direction”.
    
    In Chinese, “the way” is famously translated as tao. Christian missionaries struggling to fit Chinese attitudes into their own monotheistic outlook liked to talk about “The Tao”. But this is quite misleading, since in classical Chinese there’s often no marker for singular and plural. Instead of tao meaning “the one and only best way”, it’s simply “way”, or “ways” and there can be many ways. So a phrase like “the tao of scuba diving” is not actually silly, at least not in Chinese: it just means the way to do scuba diving. And there can be more than one.
    
    I hope you understand what I’m getting at here! I’m trying to encourage people to find a good way forward without claiming that we, the Azimuth gang, know or seek the way forward.
    
    Reply
- John Baez says:
  
  4 March, 2013 at 7:25 pm
  
  Here are some more quotes from that 1976 paper by Don Page, which I’ve managed to get now. Page works with the quantity
  
  $a_* = J/M^2$
  
  rather than Paul Davies’ rather curious quantity
  
  $a = J/M$
  
  I should mention that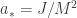 $a_* = J/M^2$ can be made dimensionless using just $G$ and $c$ , while making $a = J/M$ dimensionless requires the use of Planck’s constant. This is reflected in the fact that condition for a rotating uncharged black hole to show a naked singuarity:
  
  $cJ/GM^2 > 1$
  
  can be derived purely classically, without any quantum mechanics.
  
  This quote by Page explains that as a black hole emits Hawking radiation, $J/M^2$ goes to zero:
  
  Though one expected a black hole to give up its angular momentum in the same order of time as it gives up its mass, it has not been known whether $a_*$ tends to zero as the black hole evolves. Carter argued that it would tend asymptotically toward a fixed value less than unity, but he gave no indication of what that value would be. Numerical calculations were needed to show whether $\ln J$ always decreases faster than $\ln M^2,$ pushing $a_* = J/M^2$ toward zero, or whether these two quantities decrease equally fast at some nonzero limiting value for $a_*.$ There is some indirect evidence, to be given below, that if there were a large enough number of massless scalar fields (unknown at present and therefore not calculated in this paper) to dominate the emission, $a_*$ might indeed get hung up at some nonzero value. However, this paper shows that emission of the known massless fields can only decrease $a_*$ toward zero, and that in fact the decrease is rather rapid compared with the mass
  decrease.
  
  The actual formula for how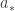 $a_*$ changes with time is complicated and depends on the species of particles we include in our Hawking radiation. But:
  
  More than half of the energy is emitted after $a_*$ is reduced below a small value, less than 0.06 for the canonical combination of species. At this point the power is within 1% of its Schwarzschild value, so the assumption that decaying black holes have negligible rotation is generally valid.
  
  I presume the ‘canonical combination’ is photons, gravitons and 3 kinds of neutrinos. (I think neutrinos were believed to be massless back then; massive particles are much less likely to be emitted at low temperatures.)
  
  This is a nice explanation of the two ‘regimes’, one where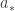 $a_*$ is big, maybe 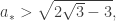$a_* > \sqrt{2 \sqrt{3} - 3},$ and one where it’s less:
  
  At high values of $a_*$ , the emission is primarily the spontaneous emission discovered by Zel’dovich that corresponds to the stimulated emission of superradiant scattering. In this process, pairs are created in the ergosphere with particles (say) being emitted to infinity with positive energies and their antiparticles going down the hole with negative energies as measured at infinity but positive energies as measured locally. In fact, the antiparticles can even be on classical trajectories at the horizon. Thus heat flows down the hole as well as out to infinity, increasing the entropy of both. On the other hand, at lower values of $a_*$ the emission is primarily thermal, drawing entropy out of the hole. The process may still be regarded as the creation of pairs, with antiparticles going down the hole having negative energies with respect to infinity, but outside the superradiant regime (which becomes negligible at small $a_*$ ), the antiparticles also have negative energy locally at the horizon and therefore cannot be on classical trajectories. Instead, they are tunneling through a classically forbidden region in virtual states that actually bring heat out of the hole.
  
  There is still some entropy produced by the partial scattering off the gravitational potential barrier surrounding the hole, but outside the superradiant regime this can only partially cancel the entropy flow out of the hole and serves in effect to increase the entropy emitted to the surrounding region for a given entropy loss by the hole.
  
  Reply
  - Cesar Uliana says:
    
    4 March, 2013 at 8:01 pm
    
    John, as you point out Page works mainly with $J/M^2$ , but the important thing to note is the fact that independently of the inital ratio, the Kerr always emits more angular momentum than mass and thus decays to a Schwarzschild configuration in the absence of accretion (which one should expect in a realistic environment). 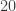Regarding the “canonical combination”, it means 1 graviton, 1 photon, 3 neutrinos and the electron, the particles with known masses below $20$ MeV (He was interested is some cosmological scenarios). So the fact that neutrinos are not really massless does not alter the discussion. Even if one is interested in large black holes where even the small neutrino mass would suppress femionic radiation the vector and tensor fields would be enough to reproduce the behavior. As I commented above the only field which could in principle decrease the mass faster than angular momentum is the scalar field, as Page points out.
    
    As for the critical value where the decay is different, Page did not know at the time of the phase transition (presumably, as he did not mention it anywhere in the paper and the first Davies paper is from the following year). The point is that for large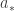 $a_\ast$ the vector and tensor fields dominate the radiaton, while for low values the spin 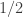$1/2$ fields have the largest contribution to the mass and angular momentum change rate. This has nothing to do with the Davies transition at $\sqrt{2\sqrt{3}-3}=0.68$ as he derived it by looking at the coefficients plotted in table I for various values of $a_\ast$ . 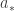Looking carefully one sees that the change in behavior occurs somewhere near $0.55$ and certainly below $0.60$ . The specific heat $C_J$ does not play any role in Hawking radiation also, as this one always decreases angular momentum. It’s importance is related to general Penrose processes where no work is done. In fact despite being more than 30 years since this phase transition showed up no one has come with a physical relevance as far as I know, though that would be very interesting. There is even a lot a of controversy regarding the critical exponents of such transition, with a handful of order parameters being suggested, but nothing seems very concrete.
    
    To the best of my knowledge the only thing which seems agreeable is that since the divergence in $C_J$ is polynomial, and not logarithmic, than it implies that the critical exponent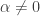 $\alpha\neq 0$ and thus this phase transition is not in the universality class of mean field theories, though I’m not sure if that idea was further explored. But I think is a nice piece of knowledge we have on the microscopical nature of black holes that people seem to ignore.
    
    Reply
  - Cesar Uliana says:
    
    4 March, 2013 at 8:03 pm
    
    John, as you point out Page work mainly with $J/M^2$ , but the important thing to note is the fact that independently of the inital ratio, the Kerr always emits more angular momentum than mass and thus decays to a Schwarschild configuration in the abscence of accretion (which one should expect in a realistic environment). 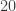Regarding the “canonical combination”, it means 1 graviton, 1 photon, 3 neutrinos and the electron, the particles with known masses below $20$ MeV (He was interested is some cosmological cenarios). So the fact that neutrinos are not really massles does not alter the discussion. Even if one is interested in large black holes where even the small neutrino mass would supress femionic radiation the vector and tensor fields would be enough to reproduce the behavior. As I commented above the only field which could in principle decrease the mass faster than angular momentum is the scalar field, as Page points out.
    
    As for the critical value where the decay is different, Page did not know at the time of the phase transition (presumably, as he did not mention it anywhere in the paper and the first Davies paper is from the following year). The point is that for large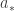 $a_\ast$ the vector and tensor fields dominate the radiaton, while for low values the spin 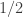$1/2$ fields have the largest contribution to the mass and angular momentum change rate. This has nothing to do with the Davies transition at $\sqrt{2\sqrt{3}-3}=0.68$ as he derived it by looking at the coefficients plotted in table I for various values of $a_\ast$ . 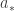Looking carefully one sees that the change in behavior occurs somewhere near $0.55$ and certainly below $0.60$ . The specific heat $C_J$ does not play any role in Hawking radiation also, as this one always decreases angular momentum. It’s importance is related to general Penrose Processes where no work is done. In fact despite being more than 30 years since this phase transition showed up no one has come with a physical relevance as far as I know, though that would be very interesting. There is even a lot a of controversie regarding the critical exponents of such transition, with a handfull of order parameters being suggested, but nothing seems very concrete.
    
    To the best of my knowledge the only thing which seems agreeable is that since the divergence in $C_J$ is polynomial, and not logarithmic, than it implies that the critical exponent 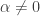$\alpha\neq 0$ and thus this phase transition is not in the universality class of mean field theories, though I’m not sure if that idea was further explored. But I think is a nice piece of knowledge we have on the mic
    
    Reply
Greg Egan says:

4 March, 2013 at 4:51 am

Here’s a plot of the temperature contours in the (M,J) plane:

The dashed line that passes through the peaks of these curves is the line of infinite $C_J$ , where energy can be added without changing the temperature (so long as it’s added in a manner that leaves J constant).

Reply
- Boris Borcic says:
  
  5 March, 2013 at 12:25 am
  
  Thanks for these perfect plots, Greg Egan.
  
  Reply
Greg Egan says:

4 March, 2013 at 8:26 am

And here’s a plot of the same temperature contours, along with the line of infinite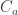 $C_a$ .

This line intersects each temperature contour at the point where the tangent to the contour passes through the origin: that is, where the tangent is a line of constant $a=J/M$ . This is where energy and angular momentum can be added to the hole in a manner that leaves $a$ constant without changing the temperature.

But it looks as if there’s nothing of particular interest about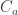 $C_a$ , because there’s no reason why any physical process would leave $a$ unchanged.

Reply
Theophanes Raptis says:

5 March, 2013 at 3:16 am

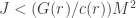Would not it been easier to assume that nature forbids such naked monstrosities by allowing the ratio $G/c$ to vary near such extremal regions so as to always have $J < (G(r)/c(r))M^2$ ? This is not unlike a restricted version of a Brans-Dicke theory.

Reply
- John Baez says:
  
  5 March, 2013 at 5:17 am
  
  Tweaking general relativity to avoid all its ‘monstrous’ solutions seems a lot less interesting than seeing where it leads. Cesar has already pointed out that the laws of black hole thermodynamics forbid gradually spinning up a black hole until it becomes extremal… just as we can’t cool something all the way down to absolute zero. If we’d merely ruled out extremal black holes by fiat, we might never have learned this.
  
  Reply
Samuel says:

5 March, 2013 at 5:28 pm

Reblogged this on Comentários, Críticas, Dicas etc.e comentado:
Very nice discussion on Rotating Black Holes.

Reply
About Supermassive Black Holes | The Arkside of Thought says:

23 March, 2013 at 7:21 pm

[…] Black Holes and the Golden Ratio (johncarlosbaez.wordpress.com) […]

Reply

	John Baez on Agent-Based Models (Part …
	Paul Pukite (@whut) on Agent-Based Models (Part …
	bhaugen on Agent-Based Models (Part …
	John Baez on Agent-Based Models (Part …
	William Waites on Agent-Based Models (Part …
	John Baez on Agent-Based Models (Part …
	John Baez on Agent-Based Models (Part …
	John Baez on Agent-Based Models (Part …
	Bruce Smith on Agent-Based Models (Part …
	William Waites on Agent-Based Models (Part …

Azimuth