Right now Kazimierz Rzążewski from the Center for Theoretical Physics at the Polish Academy of Sciences is giving a talk on “Bose statistics and classical fields”.
Abstract: Statistical properties of quantum systems are the heart of quantum statistical physics. Probability distributions of Bose-Einstein condensate are well understood for an ideal gas. In the presence of interactions only crude approximations are available. In this talk I will argue that now we have a powerful computational tool to study the statistics of weakly interacting Bose gas which is based on the so-called classical field approximation.
For a 3d ideal gas of bosonic atoms trapped in a 3d harmonic oscillator potential, the fraction of atoms in the ground state goes like
for T below a certain critical value, and 0 above that.
The grand canonical ensemble, where we assume the number of particles in our gas and its total energy are both variable, is a dubious method for Bose-Einstein condensates, because there’s no contact with a particle reservoir. The canonical ensemble is also fishy, where we assume the particle number is fixed both the total energy is variable, is also fishy. Why? Because there’s not contact with a heat reservoir, either. The microcanonical ensemble, where the energy and number of particles are both fixed, is closest to experimental reality.
We see this when we compute the fluctuations of the number of particles in the ground state. For the grand canonical ensemble, the standard deviation of the number of particles in the ground state becomes infinite at temperature below a certain value!
The fun starts when we move from the ideal gas to a weakly interacting gas. Most papers here consider particles trapped in a box, not in a harmonic oscillator — and they use the Bogoliubov approximation, which is exactly soluble for a box. This approximation involves a quadratic Hamiltonian that’s a sum of terms, one for each mode in the box. To set up this equation we need to use the Bogoliubov-deGennes equations.
As the temperature goes up, the Bogoliubov approximation breaks down… so we need a new approach.
Here is Rzążewski’s approach. A gas of bosons is described by a quantum field. But we can approximate the long-wavelength part of this quantum field by a classical field. Of course the basic idea here is not new. In our study of electromagnetism — this is what lets us approximate the quantum electromagnetic field by a classical field obeying the classical Maxwell equations. But the new part is setting up a theory that keeps some of the virtues of the quantum description, while approximating it with a classical one at low frequencies (i.e., large distance scales).
So: for modes below the cutoff we describe the system using annihilation and creation operators; for each mode above the cutoff we have 2d classical phase space. But: how to put in a nice ‘cutoff’ where we make the transition from the quantum field to the classical field?
Testing this problem on an exactly soluble model is a good idea: for example, the 1-dimensional ideal gas!
It turns out that by choosing the cutoff in an optimal way, the approximation is very good — not just for the 1d ideal gas, but also the 3d case, in both a harmonic potential and in a box. There is an analytic form for this optimal cutoff.
But more significant is the nonideal gas, where the particles repel each other. Here it’s easiest to start with the 1d case of a gas trapped in a harmonic oscillator potential. Now it’s more complicated. But we can simulate it numerically using the Metropolis algorithm!
We can also study ‘quasicondensates‘, where the coherence length is shorter than the size of the box, or the size of the cloud of atoms. (For example, in 2 dimensions, at temperatures above the Berezinskii-Kosterlitz-Thouless transition, there are lots of vortices in the gas, so the phase of the gas is nearly uniform only in small patches.)
Some papers:
• E. Witkowska, M. Gajda, and K. Rzążewski,
Bose statistics and classical fields, Phys. Rev. A 79 (2009), 033631.
• E. Witkowska, M. Gajda, and K. Rzążewski,
Monte Carlo method, classical fields and Bose statistics,
Opt. Comm. 283 (2010), 671-675.
• Z. Idziaszek, L. Zawitkowski, M. Gajda, and K. Rzążewski, Fluctuations of weakly interacting Bose-Einstein condensate, Europhysics Lett. 86 (2009), 10002.
As usual, I’d love it if an expert came along and explained anything more about these ideas. For example, I’m pretty vague about how exactly the Metropolis algorithm is used here.
Azimuth 
May non-experts ask questions?
Yeah, please do. I’m just desperately trying to get some conversations going. But you should probably do it under a pseudonym, so people don’t realize how few people are actually writing most of the comments on this blog.
Okay, but maybe it helps if you devote the first paragraph of the next edition of TWF to this blog and it’s goals.
On the other hand we don’t know if it is dis- or encouraging (or the ratio of discouraged to encouraged people) if this blog is isolated aka not overrun :-)
I certainly plan to advertise Azimuth in “week300” (last of the old series) and “week301” (first of the new).
I would like this blog to be overrun by smart scientists who energetically figure out ways of tackling the world’s big problems. I will fight to keep it from being overrun by people who like to argue in unproductive ways.
After reading your summary and browsing the references I’m somewhat confused. Path integral quantum monte carlo methods sample the exact many body density matrix at finite temperature (typically within the canonical or grand canonical ensemble). The work in the cited articles also operates in the canonical ensemble and seems to be invoking an unnecessary additional approximation wrt invocation of a classical field to simulate the ‘non condensate’. Perhaps I’m missing something.
My explanation wasn’t terribly clear, in part because I’m experimenting with live blogging, where I enter my notes from a lecture directly into a blog and do the bare minimum of post-processing. The advantage of this system is that using it, I might have the energy to create blog entries on every talk that I go to. The disadvantage is that the explanations won’t be very clear. So, people should ask questions! And ideally, experts will materialize — popping into existence like vacuum fluctuations — and answer these questions better than I can.
I’m looking at this paper:
• Emilia Witkowskaa, Mariusz Gajdaa and Kazimierz Rzążewski, Monte Carlo method, classical fields and Bose statistics, Opt. Comm. 283 (2010), 671-675.
and here’s the impression I get. Yes, in principle you can see what happens with any system in thermal equilibrium by using the Metropolis algorithm to randomly ‘walk’ around the allowed states in a way that bumps into each one with a probability proportional to
But for a cloud of hundreds or thousands of atoms this is computationally difficult. So, we need more clever tricks.
A bunch of bosonic atoms can be described by a bosonic quantum field. In this paper the trick is to treat the low-frequency vibrational modes of this field classically, while treating the high-frequency modes quantum-mechanically. This makes sense because:
1) The low-frequency modes are occupied by many atoms, while the high-frequency ones are occupied by few — that’s what happens in Bose-Einstein condensation.
2) It takes a lot more information to exactly describe a vibrational mode quantum-mechanically than classically.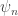
saying the amplitude for 
atoms to be in that mode.
Each mode is like a harmonic oscillator. Classically we can describe what it’s doing using just two numbers: its amplitude and phase — or if you prefer cartesian coordinates to polar coordinates, its position and momentum. Quantum-mechanically it takes a lot more information: for example, an infinite list of complex numbers
3) We can hope that the low-frequency modes behave in an approximately classical manner, and save quantum mechanics for the high-frequency modes.
As Rzążewski mentioned in his talk, the prototype of this idea is our description of light using the classical Maxwell equations. Classically, the state of each vibrational mode of light in a box is described by 2 numbers: its amplitude and phase. Quantum mechanically, what we really have in each mode is a condensate of photons! But if they’re in a coherent state, the classical description is a good approximation.
Then, the paper describes how to use the Metropolis algorithm to figure out what’s going on with the classical modes.
What about the quantum modes? I’m too tired now to extract this information from the paper — it’s not jumping out at me.
Evaluating thermal averages for 105 or more bosons is actually quite tractable with current path integral Monte Carlo methods. The basic approach goes like this:
Suppose I want the expectation value for some observable (neglecting normalization) for a many body Hamiltonian H. In the limit of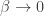
(the high temperature classical limit) there are closed form expressions for the density matrix the simplest being 
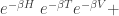error of order 
where I’m assuming a Hamiltonian of the form 
(i.e. 
kinetic energy 
and 
is a local interaction potential).
Since I only know how to treat
for small 
I need to use Feynman’s trick to write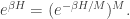 
In other words I express the low temperature (quantum) density matrix as a product of M high temperature classical ones. inserting the identity operator between each of the high temperature density matrices we have with 
,
the path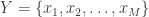
can be sampled stochastically via Monte Carlo since given two paths
and
the relative probability density is given by the
i.e. just the ratio of the products of classical density matrices over the two paths.
Even though the path Y may seem like a very high dimensional object, sampling it stochastically via Monte Carlo methods turns out to be well within current computer power since for a given statistical error the computation time only grows as the sqrt of the dimensionality of the space.
You can do LaTeX on this blog, as explained here. I changed your post to LaTeX.
Can you do 105 bosons on a PC? Kazimierz Rzążewski was talking about a PC — so it’s possible he is seeking a method that was easy to implement.
Or, it’s possible that he wants an approximation that sheds light on how we can split our description of reality into a classical part and quantum part.
A 105 particle calculation would probably take a few months on a modern PC so yes something faster that goes beyond the Gross-Pitaevskii mean field (i.e. zeroth order Bogoliubov local density approximation) could be quite useful. I’d like to get a better understanding of how well the expression for the transition between classical and quantum length/energy scales behaves as the density (interaction strength) and noncondensate fraction increase.
It would be neat if e.g. the intuitive concepts behind the two fluid model of quantum liquids could be put on a more firm footing with this approach. I wonder how it would perform in the quantum turbulence regime http://physics.aps.org/viewpoint-for/10.1103/PhysRevLett.105.045301
ps Thanks for the latex pointer. Is there a way for me to edit my previous post? It doesn’t quite make sense as it stands :)
I forget if there’s an easy way for people to edit their own posts, and I’m not sure I even want this in general, but I would love to enable some sort of ‘preview’ feature.
For now, I’ve helped Jonathan Dubois edit the LaTeX in his comment above. So, if anyone reading it still thinks it doesn’t make sense, that’s because that person needs to learn more physics. ;-)
Personally I work with many-Fermion systems, constructing many-body path integrals and performing Renormalization Group analysis. I enjoyed the run up of this thread talking about the “fishyness” of choosing an ensemble for a many-body problem. I too ran into this difficulty and have since had it “hand-waved” away from concern.
If you’re dealing with a many-body system, your Hamiltonians are undoubtedly represented in the second quantization. Since they live in the full Fock space, a proper trace means summing over all symmetrized occupation states (i.e. from vacuum up to infinite particles).
The appropriate density operator for such a system would be
. The appropriate thermodynamic potential in this case would be the “Grand Canonical Potential” 
with the number of particles given by the expression 
.
Thus you use the GC ensemble since you’re working with second quantized Hamiltonians, but the above expression for the number of particles (where
is fixed) acts as a boundary condition which can be inverted to give the chemical potential 
as a function of the temperature.