Check out this video! I start with a quick overview of network theory, and then begin building a category where the morphisms are electrical circuits. These lecture notes provide extra details:
With luck, this video will be the first of a series. I’m giving a seminar on network theory at U.C. Riverside this fall. I’ll start by sketching the results here:
• John Baez and Brendan Fong, A compositional framework for passive linear networks.
But this is a big paper, and I also want to talk about other papers, so I certainly won’t explain everything in here—just enough to help you get started! If you have questions, don’t be shy about asking them.
I thank Blake Pollard for filming this seminar, and Muhammad “Siddiq” Siddiqui-Ali for providing the videocamera and technical support.
Just finished watching the video. I’m very impressed with both the quality and the work of the camera operator following you around. (Also the ambidextrous erase old stuff with one hand and write new stuff at the same time with the other is a neat trick.) Thanks to everyone involved in making this stuff available.
A bit of a silly question: so given the existence of superconductivity (ie, real things that do have 0 resistance), how does that relate to your statement that bad things will happen when you allow 0 for one of your edge labels?
I’m sure Blake will be pleased by your comment on his camera skills. I hadn’t even known erasing with one had while writing with the other was noteworthy—though now that you mention it, it’s easier if you’re left-handed, since we write left to right.
That’s a fun physics question! Clearly a superconductor does not deliver infinite current when you put a finite voltage across it. So the question is: in what sense does it fail to have zero conductivity, and why… and why do people say it does have zero conductivity.
There are probably a number of limiting factors. Electrons can’t go faster than light, for one thing!
Excellent! What can we do to motivate you and Blake to produce further parts?
Ask questions and make comments about the lectures!
I was wondering why you define graphs as you do and not as
with 
or similar?
Instead, you make the projections
explicit and introduce ‘names’ (your set 
) for the edges just to later introduce labels, since different edges can have the same label.
There are at least 23 different things called ‘graphs’, depending on whether we
• put arrows on the edges or not
• allow multiple edges from one node to another or not
• allow edges from a node to itself or not
The logically simplest kind of graph turns out to be the one that says “yes” to all these questions: it’s a directed multigraph
which is just a pair of functions from one set to another. I say it’s “logically simplest” because this concept doesn’t really use anything about sets: we can define a directed multigraph in any category as a pair of morphisms
from some object
to some object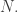 
So, when we start doing fancy stuff, it will easiest to work with this kind of graph.
I should note also that any category gives a graph of this kind, where
is the set of morphisms and 
is the set of objects. We definitely want morphisms to have a direction, and to allow more than one morphism from one object to another, and to allow morphisms from an object to itself.
For Markov processes, we don’t really need multiple edges from one node to another, or edges from a node to itself. So, none of this matters much now except that the edges must have arrows on them. But the philosophy I prefer is one where we keep things simple by not going out of our way to exclude options.
To some people this simplicity seems like complexity, but if you ever get brainwashed by category theory you’ll agree that a pair of functions
is a simpler thing than a subset
To a category theorist, the subset is a pair of functions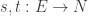
obeying the additional condition that 
is one-to-one: that is, an edge is uniquely determined by its source and target. This brings in the concept of ‘one-to-one’, or ‘monic’, making things a bit more elaborate.
Furthermore, I may sometimes want to have two roads from one city to another. So I don’t want my default notion of graph, on which I found network theory, to exclude this.
Thanks for the answer.
How mathematicians have different perspectives on the same object! For me
is (or maybe was) an abstract data type. You just tell me the properties it has and the operations I am allowed to perform. How it is constructed does not interest me the slightest ;-) Like with real numbers.
Uwe wrote:
That’s an interesting example. In the category of sets the construction of the reals using Cauchy sequences gives a result isomorphic to the construction using Dedekind cuts. But in other topoi (i.e., other categories very much like the category of sets) the Cauchy reals and the Dedekind reals can differ!
I’ve never had any need to work with real numbers in a category other than the category of sets, so this seems rather abstruse to me. However, experts tell me that the Dedekind reals behave better.
• Cauchy real number, nLab.
On the other hand, I’ve been interested in graphs in many different categories, so this is a live issue to me.
I agree completely that when working with a given structure what matters is what we can do with it, not how it was built. This is the ‘structural’ attitude that category theorists love.
One reason it pays to analyze how a structure is built is that this gives clues on when we’ll be able to build analogous structures in other contexts.
From what I said, the concept of graph that I’m using in this course will generalize from the category of sets to any category whatsoever. The kind of graph you’re talking about will generalize to any category with binary products and reasonably well-behaved subobjects.
You see, in any category we can talk about
but it takes more bells and whistles to talk about
Here we are talking about subobjects (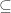
) and binary products (
). There are still tons of categories that have nicely behaved subobjects and binary products, so that’s still a huge class of contexts, but I like to leave my options open as long as possible. Of course if there were some reason I needed to use graphs of the sort 
I would do it in an instant.
For the categorically minded:
Puzzle. Using the former concept of graph, what’s a graph in the category of vector spaces? More precisely, what well-known category is equivalent to the category of graphs in the category of vector spaces?
By the way, Uwe: once upon a time we were talking about quantropy and I sort of discouraged you from studying some questions because I was feeling possessive about this idea and had ambitions of studying those questions myself. I apologize. That kind of possessiveness is almost always a dumb idea, and it was dumb in this case. I wound up getting too busy with other things to follow through on some of the questions you were starting to study!
I know in principle, but sometimes forget in practice, that it’s almost always better to encourage everyone to join in studying whatever I happen to be studying. At times someone will make so much progress that my own efforts become unnecessary. While this can be ego-damaging (and at times it’s felt terrible), the solution is for me to go do something else.
I read your pamphlets for nearly twenty years now. (Can that be true? Checking your Wiki page tells me that you started to write in 1993 which was about when I finished my phd. So yes, we are that old ;-) )
They were always **very inspiring** and **motivating** and I very much hope you **keep them coming**.
The quantropy series I liked a lot and did indeed some work on it. During that time I convinced myself that I need some serious knowledge of quantum field theory to progress further. That was beyond my resources and I shelved the project. I still think (maybe wishfull thinking) that with the ideas you have developed one can probably determine a minimal length scale for concrete quantum systems.
I think that your quantropy series was a prequel to what later became polymath projects. Your intention was different, but at least that was how I perceived it.
Thanks for the praise!
Yes, we’re getting old. The main problem with that (for me, right now) is that I feel unmotivated to re-explain things that I explained with great excitement 10 or 20 years ago. I think some people may wonder why I’m working on electrical circuits now. If I had the energy, I’d start with an explanation of why n-categories are so great, how they’re going to revolutionize our understanding of math and physics, and why we need to understand complex systems… and then I’d start talking about how to use n-categories (for n = 1 and 2) to think about electrical engineering in new ways. But I’m tired of giving the sales pitch—I want to just dive in and do the real work.
If you ever want to talk more about quantropy, let me know. Blake and I haven’t gotten around to publishing our paper on that, and while I think they’re some really exciting things left to understand, he’s moved on to other projects.
Over on G+, Daniel Estrada wrote:
I replied:
John wrote:
I would quibble with that. I would not gainsay that symmetric monoidal categories (SMCs) and operads are powereful. I am less sure that they are simple. And I am even less sure that they can tell you a lot about networks all by themselves. But perhaps you didn’t mean that.
If I were to go out on a limb, I would say there is unlikely to be one overarching theory of networks. Rather will end up with many different theories, which pay attention/model different aspects of networks. For example dynamics of networks (the network is evolving) is quite different from the dynamics on networks (a network models a dynamical system made up of interacting parts) and I have not seen anyone successfully combining these two perspectives.
The one thing I would heartily agree with John is that there is a lot of interesting mathematics carried out by scientists and engineers that applied mathematicians are largely unaware of (forget about pure). The most glaring example is hybrid dynamical systems, but there is plenty more.
Symmetric monoidal categories and operads are absurdly simple. Compare a full definition of the real numbers to a full definition of these other structures—it’s at least as long, and it’s a weird mix of ideas: algebraic structures, ordering and topology. I know because I’m teaching real analysis. “A complete ordered field” sounds short until you try to explain it from scratch… and it takes a lot of infrastructure—set theory, or second-order logic—to make sense of completeness: you’re quantifying over subsets. It takes quite a while to even construct an example!
The real numbers seem simple only because they’re familiar. We’ve all been forced to study them in school for many years… and the few people who don’t wind up failing, or hating math, find the real numbers to be simple and natural. It’s mainly just natural selection at work.
To me, category theory is less familiar but much more simple: it’s talking about the primitive abstract essences in their unadorned crystalline beauty. So in this sense (to revert to a previous conversation) I guess I am a category theorist. I really can’t think of any branch of math that’s simpler and clearer that category theory!
No, just as the real numbers all by themselves don’t say much about anything in science. These mathematical concepts are crucial if you want to say what’s going on, but you still need to have something to say.
There will be a network of theories of networks… and someday, a theory of that network.
Funny, I am teaching real analysis too this semester. So I appreciate the time it takes to explain what a complete ordered field is.
I agree that there will be a network of theories. I wonder what its classifying space looks like :)
I don’t think for continuous time dynamical systems SMCs will be enough. It’s more like presheaves of double SMCs and these presheaves are lax.
I can probably explain why “double” and it’s even easier to explain why “lax.”